42

The Meaning of Life.

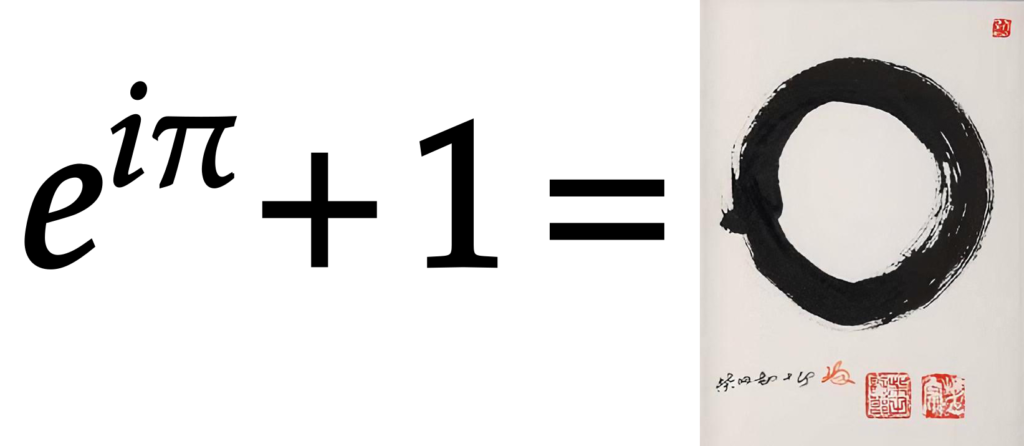
Put that on a tee shirt, put it on, and give somebody a hug. That’s the simplest way I know to express all the most important things I’ve ever learned, all at once.

You hug someone to express love for another person, consciously, emotionally and physically. That’s where the meaning of life starts and ends. Wearing the shirt while doing it symbolizes the desire to use language and symbols to share the other kinds of meaning you have discovered in your life with another human, hopefully more than one.

The stuff on the left side of the equation represents language, analysis and intellect; the right side: art, wisdom, and emotion, just like it does in our brains.

The formula is Euler’s identity, which is the most profound equation in math. To me it symbolizes truth, logic, and the beauty of abstraction. It brings together concepts from an amazingly wide range of human thought.

The image is an enso, which is maybe the simplest, most powerful symbol in Zen Buddhism. To me it symbolizes being fully present in this world in the moment, and using all the wisdom of the past to help you find your own path into the future.

First, the math part.

The simplest concept in math quantity, the simplest quantity is 1. Lots of animals can count, many can’t count very high, but if you can count, you understand what 1 means.

The simplest thing you can that requires actual math (not just counting) is addition. Multiplication (i times pi) and exponents (raising e to the i times pi) is nice snapshot of all the things humans learned about math before the calculus. The right side looks, to the left part of your brain like the number zero (which it is in Euler’s identity.) Zero is actually a fairly complicated concept that math did fine without for long periods of our history. There were written representations of “not having any” a few thousand years ago, but it was The Arabs and the Maya who independently realized that you could use it as a digit, just like 1. I think of it as the first truly abstract concept in math. That image on the right isn’t just a 0, though, it’s an enso, more on that later because it’s definitely not a math thing.

The first time I got really lost in math was trying to use Pi, and Sin and Cosine for things in trigonometry. I eventually decided that my problem was that I expected it to be logical and tidy like all math had been before, and it’s not. You can’t derive those things from first principles because they are fundamentally descriptions of the real world, not mathematical abstractions. Why is the ratio of the circumference of a circle to it’s diameter 3.1415926535… etc.? Why does that number go on forever? Why can’t I write it as a fraction? Why can’t I just do some math and figure out that Sin(pi/2) = 1, why does that have to be memorized from a table? It turns out these are just fundamental mysteries of the world as it is. Those numbers are just there for us to find. We know of no higher law that explains why that it so. And this, for me was completely unexpected, and something of a revelation – once I finally stopped banging my head against the unfairness of it all. Some things just are. You can ignore them, you can learn them, you can get them right or wrong, but getting them right is always an approximation.

Humans have struggled with understanding the difference between round an square, as quantized by PI for a very long time. One of the longest lived math challenges in history was the problem of “squaring the circle.” The question asked by the ancient Greeks some 2,500 years ago was: “how can you use just a compass and a straight edge to take a circle, and construct a square with exactly the same area?” In 1882, It was finally proven to be completely impossible because PI isn’t just irrational, it’s “transcendental” (it cannot be a solution of an equation involving only finite sums, products, powers, and integers.) It’s transcendent be exactly right about the value of PI, you need to get to infinity, which can never happen. Does this mean that infinity is real in the world, because real things have infinities buried in our explanation of them? I don’t think so, but nobody actually knows things like that. If you need to calculate round things, or, as it turns out, periodic things, you have to approximate a value for PI. So in practice, you just decide how much you care about the details and go with something that’s close enough. Sound familiar?

e is Euler’s number. It’s irrational, like PI, as far as we know the digits keep going on forever. Also like PI, you can calculate it if you work on it for an infinitely long time, which of course you can’t, so you have to use an approximation again. It’s the simplest infinite series I know that’s actually useful (and e is fantastically useful.) It’s 1 + 1/2 + 1/3 + 1/4 + … + 1/infinity, which works out to 2.7182818284… etc. It’s probably the most useful constant in science. Biologists and economists use it to model the particular kinds of growth they are interested in. Calculus depends on it very heavily to solve differential equations with exponents in them; probability calculations lean hard on it, too, and physics needs to do those sorts of thing all the time.

i is the square root of negative one, and it is the weirdest of them all. It is also one of the most pivotal concepts in the transformation of modern society because it unlocked the mysteries of quantum mechanics, without which you’d need a crew and a u-haul to move you computer, and it would have only a fraction of the power of the free game in your Happy Meal. It turns out that this weird thing, i, invented to make more elegant solutions to problems of pure math, is just the thing to make the Schrodinger Wave equation work, which is in turn used to predict the behavior of virtually everything else in our universe except gravity, and a few very interesting other things that I will get to soon.

The fact that you can put all these gigantic concepts into one, tiny, meaningful, true equation is incredibly beautiful if you look at it right. It just seems so undeniably deep, somehow. I am far from the first person to be inspired to relate it to all the other important things in their life. If I were a religious man, I would have invoked God in the first sentence.

Now the not-math part.

On my tee shirt, it’s not a zero on the right side, it’s an enso, which is a symbol from Zen Buddhism. It is the most clear, and concise expression of Zen that I know, because it generally means exactly what you think it does when you first see it, and continues to feel that way no matter how deeply you contemplate it. For me it’s a well from which I could potentially draw whatever I need, and a window through which I can see the whole of existence from the farthest reaches of the galaxy to the deepest, most secret corners of my soul. But why?

Basically, it just means circular. It’s linguistic roots go back to a Chinese character originally depicting the top of a pot, round, and one originally meaning “eyeing a tree,” the shape of a thing. Since then, it’s come to mean a lot more. It means nothing, because the circle is empty. It means everything, just like when you wave your hand in a circle to imply “the whole shebang.” It means a lot more, too, as you might imagine from a symbol that can simultaneously mean everything and nothing. The first enso we know about was drawn by a Chan Buddhist monk about 1,200 years ago. When Chan got to Japan it became Zen, which I understand better, so to me the enso is a Zen thing, even though, like almost everything else in that part of the world, it came from China originally.

Calligraphy is the recording of a moment in time, pure and unfiltered. You practice it with single-minded focus until you can dip the brush, and in one, fluid stroke, get the desired effect. You never fix it, you just frame the really good ones and toss the rest. You keep the good ones because it helps you, and hopefully others understand the benefits of mindfulness. By appreciating the subtle flow of really good calligraphy; how alive it looks, how it freezes time so you can almost be there, in the room where it was painted, you get the experience of learning from a master you have never met. The enso is the simplest interesting character you can paint. Its shape is easy to understand and hard to get perfect, like so much in life. Its meaning is just as simple, just as profoundly complex, and ultimately, just as intuitive. It is perfect both as the first exercise for a beginner, and as the culmination of a lifetime of practice. Which is just the kind of circle of life that the enso represents. I found a really good description of all the things that the enso symbolizes here: https://modernzen.org/enso-htm/

There is a tension in calligraphy, though. What matters is one-pointed attention and spontaneity in the act of producing it, but then you hang it on the wall to look at when you want to think about things instead of doing them. And traditionally, it’s a famous person’s work, not your own. What’s up with that? The answer is compassion. The thing that made Siddhartha Buddha different from all the people who “achieved enlightment” before, is that he wanted to rejoin the world to help other people learn to experience what he experienced. That’s why you hang it on the wall. Because the best and most valuable things people learn, are learned best by face-to-face communication with somebody that knows them.

But oral traditions can get garbled, so how do you preserve the inspirations of the few humans who get really good ideas, without getting distracted by the thing that you are passing down through the generations? Most traditions get this wrong, in my opinion. Zen and Taoism get it right. You make the attempt, because it’s important, but you do everything you can to make sure subsequent generations understand that what you’re really trying to do is get them into the same, particular state of mind that the teacher achieved, because that is always the thing most worth knowing, not the words they used trying to describe it to their disciples.

The enso symbolizes this truth in a couple ways. The first description of it was something like “A vast empty space, lacking nothing and containing nothing extraneous.” This is the state of mind you want to bring about when you meditate. A koan is a little, Zen word game designed to help you let go of your attachment to ordinary ways of thinking. The one everyone knows is “what is the sound of one hand clapping.” The first one they teach you is just “mu.” Which means nothing, literally. You are trying to achieve complete focus on something that means nothing, just as you are when drawing, or looking at a perfect enso. When you meditate, you empty your mind of extraneous things. That creates space for you to expand your awareness of everything else and directly experience the interconnected of all things. It’s a symbol of that process. It also looks like the moon, which is a symbol of enlightenment, and the source of my favorite little bit of Zen wisdom: don’t get fixated on my finger, look where it’s pointing and see for yourself. Words are useless if they don’t lead you to have the experience I’m trying to share with you. Lao Tzu knew this. The first words of the Tao te Ching (The way of Change) is “The Tao that can be spoken is not the true Tao.” Since his words were spoken and later written down by his students, that means the first thing out of his mouth was basically “ignore what comes out of my mouth.” But of course you aren’t meant to ignore it, just take the words that help and shrug off the ones that don’t. Just like you should do with all religion, philosophy, and the haters in your comments section.

Now the philosophy of science part.

The reason I replaced the zero in Euler’s identity with the enso symbolizes the synergy I found between the ultimate flowering of Ancient Eastern philosophy and cutting edge modern science. Everywhere I look, I see that parallel, and the enso is a fitting symbol for most of it. What is quantum entanglement and Quantum Field Theory besides the interconnectedness of all things? Take Yin/Yang. At it’s heart, it’s the intuition that not only does everything we encounter come to us as two sides of a single coin, but that opposites are so alike, intrinsically, that one has the other at its heart, and in fact, they both arise from the same original unity. In physics, new particles are created all the time, and they like to do it in pairs. If you try to pull two quarks apart, you never get a two, isolated quarks. Instead, when they get to just the right distance, they use all that energy you spent pulling them apart to create a new quark/antiquark pair in between.

Then there’s cosmology. The biggest problem with our theory of everything is that it isn’t the one, grand, unified theory (GUT) that we want it to be. We want it partly because of how cool THAT equation would be, but mostly because there’s some very troubling data that obviously mean we’re missing something, probably something so big that if one person just figured it out, they would instantly become “The New Einstein.” What we do have is kind a duality of what we know about the unimaginably small (Quantum Field Theory or QFT,) and the really big (General Relativity.) They mix like bleach and ammonia – killing the hopes of whoever tries to get something done by putting them together. The enso symbolizes this tension for me at many levels. We can feel in our gut that everything is connected, so why shouldn’t that be describable with one set of rules that describes how everything works, everywhere? That’s mostly an esthetic judgement, truth is pretty, and a single truth of everything is the most beautiful idea we can imagine. But where did we get our sense of beauty if not from the world? Maybe it’s a perfectly valid intuition. I think it’s the same intuition expressed by creating, in one, fluid, perfect moment of brushwork, everything and nothing at the same time. It’s beautiful.

One of the problems things you could resolve that would get people calling you “The New Einstein” is referred to by cosmologists as the Hubble Tension. It’s a discrepancy between two ways of measuring the expansion of the universe that, according to everything we know about everything, should both work equally well and come to the same answer. Earlier this month (March, 2024,) a study was published triple-checking the data and leaving no doubt that we’re missing something because everything we know about everything very confidently predicts, with great accuracy and precision, that the universe is expanding at two different speeds at once; not one speed in one place, and another somewhere else, everywhere. There’s something very wrong with everything we know about everything and we have no idea what it is, except that it’s something we don’t know about nothing. That is, “empty” space is doing something weird and we have no idea what it is.

You’ve heard of Dark Matter, and Dark Energy? They were imagined in order to solve similar problems with data discrepancies showing us weird things about empty space. Dark matter is extra mass you need to have spinning around galactic centers to explain why the stars at the edge aren’t moving a lot slower than the ones in the middle. Imagine a bunch of toy boats getting sucked down a big drain in the middle of a swimming pool. The ones in the middle whip around really fast and the ones near the edge are barely moving. Trouble is, all the mass we can see in galaxies, stars and gasses emitting light and dust absorbing it, moves around the center much too close to the same speed. Dark matter is anything that has mass, but we can’t see. The math doesn’t really support it being just little bits of regular matter that’s hard to see, so they are looking for a new kind of particle which doesn’t interact with the electomagnetic force at all, like neutrinos. But not neutrinos, because we already know there are already a stupefying number of them passing through our bodies every second, we know why there are that many, and they are too tiny to be of any help unless there’s something really wrong with the equations that work so well for everything else we can measure. So the solution is, empty space isn’t empty, it’s full of something very mysterious, which we’re going to call Dark Matter because we’re looking for something entirely new that has mass and doesn’t interact with light, so what else would you call it?

Dark Energy is the strange one. When Einstein was formulating his famous theory, smart people all believed in a “Steady State” universe, that is, that the universe was always like it is, and always would be. But his equations said that all the mass should be pulling spacetime together, collapsing the universe. To fix it, he invented a Cosmological Constant to jam into his equation to push spacetime apart exactly enough to perfectly balance the inward force of gravity. Then the Cosmic Microwave Background was measured and it convinced everyone that what had been scorned as the ridiculous “Big Bang” theory, was in fact correct. The universe was expanding. Einstein was chagrined, calling his Cosmological Constant “my greatest blunder.” Then telescopes got better and data didn’t agree with the theory again. Turns out, if relativity and QFT are right, there IS something pushing the universe apart. It’s expanding faster than it should. They were even more in the dark about this cause of new discrepancy than they were about the galactic mass issue, so they called whatever-it-was Dark Energy for now, and got on with figuring out how hard it was pushing. The theory goes that empty space has something in it that works like anti-gravity. In fact, there’s really no reason I know of why you couldn’t call Dark Energy that. Maybe I will from now on and see if anybody can tell me what’s wrong with that.

Now my favorite bit, where I draw even more questionable conclusions than Dark Energy = Antigravity and decide to believe them just because I think they’re pretty.

QFT basically tells us that everything in the world is made of nothing. That is to say, physical things aren’t really things at all, in the literal sense, they are vibrations in a field (well, ok, in a few fields) spanning all of the universe in all directions. Solid objects are really more like three dimensional ripples on pond. If the world is the surface of a pond, ripples are very important, but are they a separate thing in their own right? Or, to paraphrase Gary Zukav’s amazing1979 book, The Dancing Wu Li Masters, are they just one particular way the world wiggles while it dances? Is space really just the giant orchestra of the universe, and everything in it just the music that it’s playing? I’m going to call this my “Big Band Theory” from now on.

Which brings me, finally, to me. Specifically, what do I mean when I say “I.” More formally, to my theory of mind and the Hard Problem of Consciousness. Descarte gave us “cogito ergo sum,” which isn’t a terrible place to start. I look for myself in the music that my brain and body is playing (in a corollary to my Big Band Theory,) which is a pretty similar thing to say when you get right down to it. To me, the only really important difference between my bodymind music and rock music (not The Rolling Stones, ACTUAL stones) is that I am conscious, and rocks aren’t. So what’s that mean? The only story about that I’ve ever heard that sounds promising to me is the one Sir Rodger Penrose tells. He started as a maths guy, got interested in relativity and Quantum Mechanics at Oxford, and got really disturbed by Gödel’s incompeletness incompleteness theorem, which essentially states that no consistent formal system can prove its own consistency. The way Penrose puts it is that if you believe that any set of rules really works there will always be something you believe that the system cannot prove. What it told him was that consciousness was non-computational. Then he invented some things back in ’74 that we now call Penrose Tiles that quickly illustrate his point in a fairly incontrovertible way. Interestingly, they have very recently shown promise as the basis for quantum-proof cryptograph, but while I think it’s connected, I also think that EVERYTHING is connected, so I’ll leave that story for another day. He then went casting about for something consciousness could be made of that wasn’t computational and could be found in the brain. He decided he was looking for quantum behavior buy couldn’t find anything in neurons and synapses that could do the job. So he put it in his excellent book, The Emperor’s New Mind, and went about his usual business of being one of the smartest guys alive until an anasthesiologist name Stuart Hammeroff told him about micro-tubules and he got all excited. Then I saw him talk about it and he got me all excited. Here’s a place where it might work, but first, some more background.

Sir Penrose has gotten increasingly rude about quantum mechanics as he reached for his ninth decade. He used to demurely agree with the pioneers of the theory that it was simply incomplete, but now he says it’s broken. It’s the same GUT feeling. Gravity and QFT don’t mix. But he points to the exact place where the problem lies. The collapse of the wave function. Schrödinger’s Cat is a thought experiment to show you the problem, quantum mechanics predicts that the cat is both a certain alive and dead at the same time. Everybody knows the world doesn’t work like that, but the math so perfectly predicts so many amazing things about how the world DOES work that everyone just kind of bleeps over the problem and goes to work anyway. Even worse, relativity completely self-destructs when you try to let superpositions like that happen, that’s why GUTs don’t work.

So what is the core problem, really? Ok, QFT requires some kind of interaction from outside a quantum system to cause the system to pick one or the other of two states in superposition, according to VERY well defined rules of probability. But mass bends spacetime and you absolutely cannot make the equations make any sense at all if you allow a massive particle to try to bend spacetime from two different places at once. The wave that the function is talking about describes the amplitudes of probability that a particle would be found in one place or another if you were to measure it. Those waves cancel and reinforce each other in very counter-intuitive ways, but it always works in simple systems which are small enough to ignore mass. You get to ignore mass because the force of gravity is so very tiny compared to the other forces of nature. Measuring the system “collapses” the wave function, choosing one particular outcome over the other. If you do it a million times, you see the interference patterns predicted by the wave equation. But if you don’t do it at all, the cat is still both alive and dead. Which is absurd.

Penrose’s solution to that problem is so very simple and elegant in concept, but way too rigorously mathematical for me to even understand in detail, let alone explain. Suffice it to say that, before an actual particle with mass gets very far (around the Plank length, which is unimaginably small, and completely impossible to ever measure directly. 1.616255×10−35 meters) the universe itself somehow picks one or the other and the wave function is reduced objectively, not because it was subjected to any external influence, so he calls this process Objective Reduction.

Now it gets interesting.

What if this is the mysterious quantum process that consciousness is built out of? It’s a real, physical process (if it exists,) but it’s definitely non-computational. What if everything in the universe is participating in tiny moments of “proto-consciousness” all the time by picking which branch of the wave function survives collapse? Even cooler, what if you can orchestrate all those little moments to create all kinds of amazing emergent phenomenon? He calls his theory Orchestrated Objective Reduction, Orch-OR for short.

You can run a bunch of ones and zeros through a calculator and end up with Chat-GPT, so maybe you can combine a trillion wave function collapses in just the right way and end up with Einstein? This would mean that the thing that makes me me is that my body has drawn a circle around empty space and coordinated the crazy things that happen there in when the nothingness turns into something. All of that something inside me chugs along, quietly choosing it’s path through my body, and my consciousness conducts the whole orchestra, deciding my path around the circle of life.

But this isn’t a tee-shirt hug, it’s an explanation, which is already wide of the point and almost as pretentious as the title of one of my big papers in college: “Existential and Ontological Dimensions of Time in the Works of Heidegger and Dōgen.” I giggled when I wrote it, and it still makes me chuckle now. That’s why the title and the subtitle are drawn from the works of my favorite comedians. Because maybe the most important thing to learn is: be nice to people, think about cool stuff, but don’t take yourself too seriously or the immortal Lao Tzu just might appear and bite off your nose!